How To Find Gcf With Prime Factorization

Every whole number greater than 1 has a prime factorization — that is, the list of prime number numbers (including repeats) that equal that number when multiplied together. For example, here are the prime factorizations of 14, 20, and 300:

14 = two × vii

20 = 2 × 2 × v

300 = 2 × 2 × 2 × three × five

Gene copse are a useful tool for finding the prime number factorization of a number. For case, to find the prime factorization 30, first find a gene pair — a pair of numbers that, when multiplied together, equals thirty:

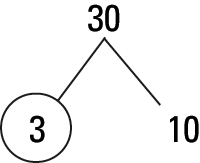
Annotation that 3 is circled because it'due south a prime. But 10 is not prime, so you tin can continue the tree, finding a factor pair for 10:

This time, both ii and 5 are circled considering they're each prime number numbers. When the bottom numbers on the factor tree are all prime, you take your answer: 30 = 2 × 3 × 5.

Using prime factorization to find the GCF

You can use prime number factorization to observe the greatest common factor (GCF) of a set of numbers. This method often works ameliorate for large numbers, when generating lists of all factors can be fourth dimension-consuming.

Here's how to find the GCF of a fix of numbers, using prime factorization:

Listing the prime factors of each number.
Circumvolve every mutual prime factor — that is, every prime factor that's a factor of every number in the set.
Multiply all the circled numbers.

The result is the GCF.

For example, suppose yous want to discover the GCF of 28, 42, and 70. Step one says to list the prime factors of each number. Step ii says to circle every prime factor that's common to all three numbers:

Equally yous tin can run into, the numbers ii and 7 are mutual factors of all iii numbers, so multiply these two numbers as follows:

2 × 7 = 14

Thus, the GCF of 28, 42, and lxx is 14.

Knowing how to detect the GCF of a set of numbers is important when yous begin reducing fractions to lowest terms.

Using prime factorization to find the LCM

One method for finding the to the lowest degree mutual multiple (LCM) of a set of numbers is to use the prime factorizations of those numbers. Here'southward how:

List the prime factors of each number.

Suppose yous want to notice the LCM of xviii and 24. Listing the prime factors of each number:

eighteen = 2 × iii × 3

24 = 2 × 2 × 2 × 3
For each prime number number listed, underline the most repeated occurrence of this number in whatsoever prime factorization.

The number 2 appears one time in the prime factorization of xviii but three times in that of 24, and then underline the three 2s:

xviii = ii × three × 3

24 = 2 × two × 2 × 3

Similarly, the number 3 appears twice in the prime factorization of 18 simply only once in that of 24, so underline the two 3s:

18 = 2 × 3 × three

24 = ii × 2 × two × three
Multiply all the underlined numbers.

Here'southward the product:

2 × ii × 2 × 3 × 3 = 72

So the LCM of xviii and 24 is 72. This solution checks out considering

18 × four = 72

24 × 3 = 72